1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
|
clear all; close all;
a=1;
T=1;
h=0.0025; x=[0:h:1];
tau=0.0025; t=[0:tau:T];
r=a*tau/h;
M=length(x)-1; N=length(t)-1;
[T X]=meshgrid(t,x);
e=r^2*ones(M-1,1);
A=spdiags([e 2*(1-e) e],[-1 0 1],M-1,M-1);
u=zeros(M+1,N+1);
u(:,1)=sin(4*pi*x');
u(:,2)=sin(4*pi*x')+tau*sin(8*pi*x');
u(1,:)=0; u(end,:)=0;
for n=2:N
u(2:M,n+1)=A*u(2:M,n)-u(2:M,n-1);
u(2,n+1)=u(2,n+1)+r^2*u(1,n);
u(M,n+1)=u(M,n+1)+r^2*u(end,n);
end
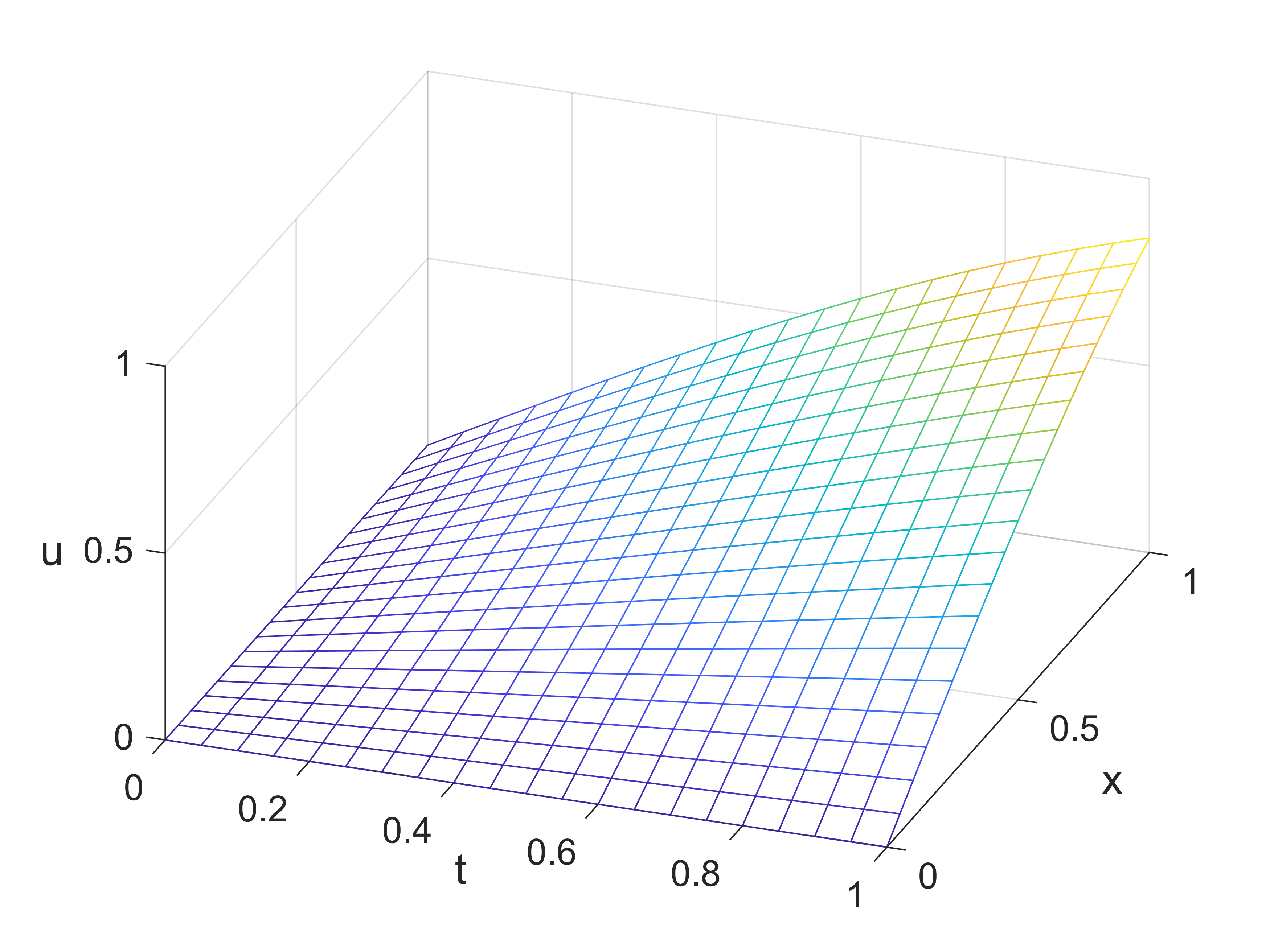
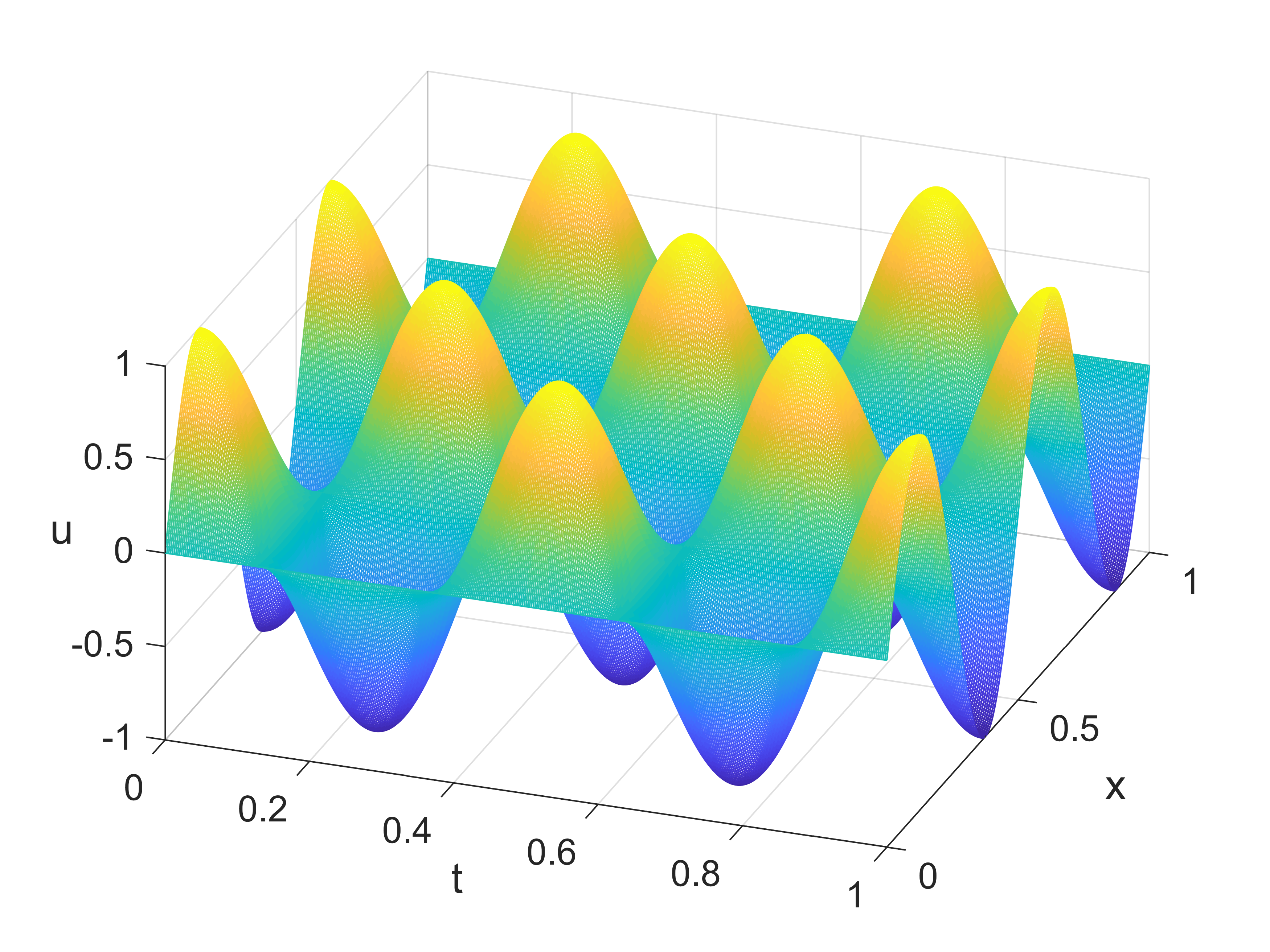
mesh(t,x,u), view(20,40)
set(gca,'fontsize',12)
xlabel('t','fontsize', 14)
ylabel('x','fontsize',14)
zlabel('u','fontsize',14)
ue=sin(4*pi*X).*cos(4*pi*T)+sin(8*pi*X).*sin(8*pi*T)/(8*pi);
disp('t = 1, 2, 3, 4, 5')
Error=max(abs(ue(:,1/tau+1:1/tau:end)-u(:,1/tau+1:1/tau:end)))
|